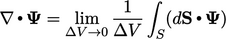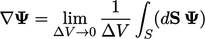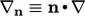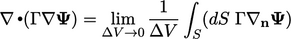Notes on Computational Fluid Dynamics: General Principles

2.23 Summary of equations

• Force 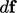at a surface:
  
• Rate of deformation

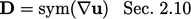
Conservation laws

• Mass, Sec. 2.4
• Momentum, Sec. 2.7
• Energy, Sec. 2.15

Constitutive models

• Newtonian fluid, Sec. 2.12
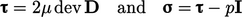
• Fourier’s law, Sec. 2.16
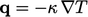
• Ideal gas, Sec. 2.16
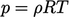
• Specific heat capacity, Sec. 2.18
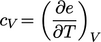
• Energy-temperature relation, Sec. 2.19

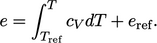
Derivatives

• Material time derivative, Sec. 2.5
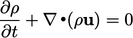
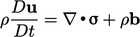
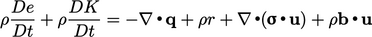
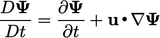
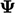• Divergence, Sec. 2.4 , is vector, tensor
• Gradient, Sec. 2.12
• Laplacian, Sec. 2.14 ,